Page 24 - EngineerIT March 2022

MEASUREMENT

Governing equation Table 2. Young’s Modulus (E), Shear Modulus (G), Density (ρ), and Cost
While FEM provides significant benefits in solving the per kg of Common Industrial Metals
Timoshenko equation of vibration in an efficient manner, an 2 2 3
understanding of the trade-offs in designing a vibration sensor Material E (N/m ) G (N/m ) ρ (kg/m ) $ per kg
2
enclosure requires closer examination of the equation 4 Stainless steel 2E11 7.7E10 7850 0.11
parameters. Copper 1.1E11 4.5E10 8300 9.06
Aluminum 7.1E10 2.4E10 2770 2.18
Titanium 9.6E10 3.6E10 4620 25

Using different materials or geometries will affect the natural What material should I use for my design?
frequency (ω) of the designed structure. Table 2 details some common industrial metallic materials such as
stainless steel and aluminum.
Material and geometry dependencies Copper is the heaviest material of all four listed, and it doesn’t
The Timoshenko equation parameters can be grouped as either provide any advantage over stainless steel, which is lighter,
geometry dependent or material dependent. stronger, and less expensive.
Aluminum is a good choice for weight sensitive applications. Its
Material dependencies are: density is 66% less than steel. The downside is that aluminum costs
• Young’s modulus (E): this is a measure of the elasticity of a 20× steel per kilogram. Steel is the clear choice for cost sensitive
material – how much tensile force is required to deform it. A applications.
tensile deforming force occurs at right angles to a surface. Although titanium is about two-thirds heavier than aluminum,
• Shear modulus (G): this is a measure of the shear stiffness of a its inherent strength means that you need less of it. However, using
material – the ability of an object to withstand a shear stress titanium is cost prohibitive for all but the most specialised weight
deforming force when applied parallel to a surface. saving applications.
• Material density (ρ): mass per unit volume.
Simulation Example
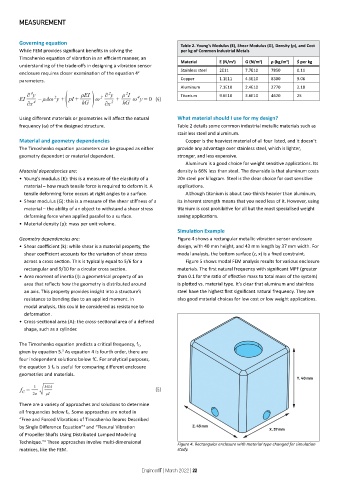
Geometry dependencies are: Figure 4 shows a rectangular metallic vibration sensor enclosure
• Shear coefficient (k): while shear is a material property, the design, with 40 mm height, and 43 mm length by 37 mm width. For
shear coefficient accounts for the variation of shear stress modal analysis, the bottom surface (z, x) is a fixed constraint.
across a cross section. This is typically equal to 5/6 for a Figure 5 shows modal FEM analysis results for various enclosure
rectangular and 9/10 for a circular cross section. materials. The first natural frequency with significant MPF (greater
• Area moment of inertia (I): a geometrical property of an than 0.1 for the ratio of effective mass to total mass of the system)
area that reflects how the geometry is distributed around is plotted vs. material type. It’s clear that aluminum and stainless
an axis. This property provides insight into a structure’s steel have the highest first significant natural frequency. They are
resistance to bending due to an applied moment. In also good material choices for low cost or low weight applications.
modal analysis, this could be considered as resistance to
deformation.
• Cross-sectional area (A): the cross-sectional area of a defined
shape, such as a cylinder.

The Timoshenko equation predicts a critical frequency, f C,
given by equation 5. As equation 4 is fourth order, there are
3
four independent solutions below fC. For analytical purposes,
the equation 5 f C is useful for comparing different enclosure
geometries and materials.

There are a variety of approaches and solutions to determine
all frequencies below f C. Some approaches are noted in
“Free and Forced Vibrations of Timoshenko Beams Described
by Single Difference Equation” and “Flexural Vibration
3
of Propeller Shafts Using Distributed Lumped Modeling
Technique.” These approaches involve multi-dimensional Figure 4. Rectangular enclosure with material type changed for simulation
4
matrices, like the FEM. study.

EngineerIT | March 2022 | 22